Questions about surgery theory
This page organizes questions and answers about surgery theory.
The natural first port of call for quick answers is Mathoverflow.
Below is a list of questions, possibly with answers.
The Atlas also has a chapter Questions for questions which attract longer answers.
Contents |
1 Questions
1.1 How can you tell if a space is homotopy equivalent to a manifold?
This is in fact a Mathoverflow question.
1.2 Simply connected surgery obstruction groups
How does one prove that
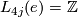 ,
, 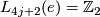 and
and 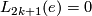 ?
?
Read [Kervaire&Milnor1963] and/or [Browder1972] and/or [Ranicki2002, Chapter 12].
1.3 CW structures on topological manifolds
Topological manifolds of dimension 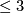 have a piecewise lienar (in fact a differentiable)
structure, and a fortiori are triangulable. Topological manifolds of dimension
have a piecewise lienar (in fact a differentiable)
structure, and a fortiori are triangulable. Topological manifolds of dimension 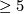 have a handlebody
structure, and hence a
have a handlebody
structure, and hence a  structure; it is still not known whether there exist non-triangulable
examples. It is known that there exist non-triangulable topological 4-manifolds, such as the Freedman
structure; it is still not known whether there exist non-triangulable
examples. It is known that there exist non-triangulable topological 4-manifolds, such as the Freedman
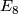 -manifold. Do topological 4-manifolds have a
-manifold. Do topological 4-manifolds have a  structure?
structure?
2 References
- [Browder1972] W. Browder, Surgery on simply-connected manifolds, Springer-Verlag, New York, 1972. MR0358813 (50 #11272) Zbl 0543.57003
- [Kervaire&Milnor1963] M. A. Kervaire and J. W. Milnor, Groups of homotopy spheres. I, Ann. of Math. (2) 77 (1963), 504–537. MR0148075 (26 #5584) Zbl 0115.40505
- [Ranicki2002] A. Ranicki, Algebraic and geometric surgery, The Clarendon Press Oxford University Press, Oxford, 2002. MR2061749 (2005e:57075) Zbl 1003.57001