Pin structures
(Created page with "<!-- COMMENT: To achieve a unified layout, along with using the template below, please OBSERVE the following: besides, $...$ and $$...$$, you should use two environments: - Fo...") |
|||
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{{Stub}} | {{Stub}} | ||
== Introduction == | == Introduction == | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
<wikitex>; | <wikitex>; | ||
For an oriented manifold a [[Wikipedia:Spin_structure|spin structure]] is a reduction of the structure group of its | For an oriented manifold a [[Wikipedia:Spin_structure|spin structure]] is a reduction of the structure group of its | ||
| − | |||
tangent bundle from the connected topological group $SO(n)$ to the double (universal) cover $Spin(n)$. | tangent bundle from the connected topological group $SO(n)$ to the double (universal) cover $Spin(n)$. | ||
The non-connected group $O(n)$ has two nontrivial central extensions (double covers) by $\Zz_2$ | The non-connected group $O(n)$ has two nontrivial central extensions (double covers) by $\Zz_2$ | ||
| Line 31: | Line 8: | ||
A $Pin^+$-structure on a manifold is thus a a reduction of the structure group of its tangent bundle | A $Pin^+$-structure on a manifold is thus a a reduction of the structure group of its tangent bundle | ||
from $O(n)$ to $Pin^+(n)$, and similarly for $Pin^-$-structures. | from $O(n)$ to $Pin^+(n)$, and similarly for $Pin^-$-structures. | ||
| + | |||
| + | A $Pin^+$-structure on $M$ is equivalent to a $Spin$-structure on $TM\oplus 3 det(TM)$, | ||
| + | a $Pin^-$-structure on $M$ is equivalent to a $Spin$-structure on $TM\oplus det(TM)$. | ||
The obstruction for existence of a $Pin^+$-structure on $M$ is the characteristic class $w_2(M)$. | The obstruction for existence of a $Pin^+$-structure on $M$ is the characteristic class $w_2(M)$. | ||
| − | If $M$ does admit $Pin$ structures, then the set of isomorphism classes of $Pin^+$-structures on $M$ is | + | If $M$ does admit $Pin$ structures, then the set of isomorphism classes of $Pin^+$-structures on $M$ is acted upon freely |
| + | and transitively by $H^1(M;\Zz_2)$. | ||
The obstruction for existence of a $Pin^-$-structure on $M$ is the characteristic class $w_2(M)+w_1(M)^2$. | The obstruction for existence of a $Pin^-$-structure on $M$ is the characteristic class $w_2(M)+w_1(M)^2$. | ||
| − | If $M$ does admit $Pin$ structures, then the set of isomorphism classes of $Pin^-$-structures on $M$ is | + | If $M$ does admit $Pin$ structures, then the set of isomorphism classes of $Pin^-$-structures on $M$ is acted upon freely |
| − | + | and transitively by $H^1(M;\Zz_2)$. | |
A $Pin^\pm$-structure together with an orientation is equivalent to a $Spin$-structure. | A $Pin^\pm$-structure together with an orientation is equivalent to a $Spin$-structure. | ||
| + | There is also a group $Pin^c$ which is a central extension of $O(n)$ by $S^1$. | ||
| + | $Pin^c$-structures are obstructed by the integral characteristic class $W_3(M)$, | ||
| + | and if they exist, isomorphism classes of $Pin^c$-structures are in bijection with $H^2(M)$. | ||
| + | For more information on $Pin$-manifolds, including a computation of the low-dimensional bordism groups | ||
| + | of $Pin$-manifolds, see {{cite|Kirby&Taylor1990}}. | ||
</wikitex> | </wikitex> | ||
== Examples == | == Examples == | ||
<wikitex>; | <wikitex>; | ||
| + | In dimension $2$, all orientable surfaces admit a $Spin$-structure, and hence both $Pin^+$- and $Pin^-$-structures. The nonorientable surface $N_n := \#^n \RP^2$ admits a $Pin^-$-structure, but admits a $Pin^+$-structure if and only if $n$ is even. | ||
| + | |||
For $k\ge 1$: | For $k\ge 1$: | ||
$\RP^{4k}$ admits two $Pin^+$-structures and no $Pin^-$-structure. | $\RP^{4k}$ admits two $Pin^+$-structures and no $Pin^-$-structure. | ||
| + | |||
$\RP^{4k+1}$ admits no $Pin^+$-structure and no $Pin^-$-structure. | $\RP^{4k+1}$ admits no $Pin^+$-structure and no $Pin^-$-structure. | ||
| + | |||
$\RP^{4k+2}$ admits no $Pin^+$-structure and two $Pin^-$-structures. | $\RP^{4k+2}$ admits no $Pin^+$-structure and two $Pin^-$-structures. | ||
| + | |||
$\RP^{4k+3}$ admits two $Pin^+$-structures and two $Pin^-$-structures. | $\RP^{4k+3}$ admits two $Pin^+$-structures and two $Pin^-$-structures. | ||
</wikitex> | </wikitex> | ||
Latest revision as of 21:22, 2 July 2011
|
This page has not been refereed. The information given here might be incomplete or provisional. |
[edit] 1 Introduction
For an oriented manifold a spin structure is a reduction of the structure group of its
tangent bundle from the connected topological group 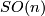 to the double (universal) cover
to the double (universal) cover 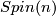 .
The non-connected group
.
The non-connected group 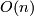 has two nontrivial central extensions (double covers) by
has two nontrivial central extensions (double covers) by  with different group structures, denoted by
with different group structures, denoted by 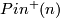 and
and 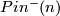 .
A
.
A 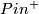 -structure on a manifold is thus a a reduction of the structure group of its tangent bundle
from
-structure on a manifold is thus a a reduction of the structure group of its tangent bundle
from 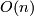 to
to 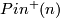 , and similarly for
, and similarly for 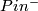 -structures.
-structures.
A 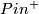 -structure on
-structure on  is equivalent to a
is equivalent to a  -structure on
-structure on 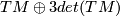 ,
a
,
a 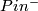 -structure on
-structure on  is equivalent to a
is equivalent to a  -structure on
-structure on 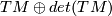 .
.
The obstruction for existence of a 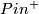 -structure on
-structure on  is the characteristic class
is the characteristic class 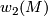 .
If
.
If  does admit
does admit 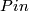 structures, then the set of isomorphism classes of
structures, then the set of isomorphism classes of 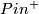 -structures on
-structures on  is acted upon freely
and transitively by
is acted upon freely
and transitively by 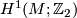 .
.
The obstruction for existence of a 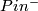 -structure on
-structure on  is the characteristic class
is the characteristic class 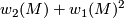 .
If
.
If  does admit
does admit 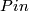 structures, then the set of isomorphism classes of
structures, then the set of isomorphism classes of 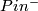 -structures on
-structures on  is acted upon freely
and transitively by
is acted upon freely
and transitively by 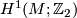 .
.
A 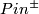 -structure together with an orientation is equivalent to a
-structure together with an orientation is equivalent to a  -structure.
-structure.
There is also a group 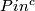 which is a central extension of
which is a central extension of 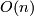 by
by  .
.
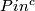 -structures are obstructed by the integral characteristic class
-structures are obstructed by the integral characteristic class 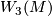 ,
and if they exist, isomorphism classes of
,
and if they exist, isomorphism classes of 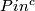 -structures are in bijection with
-structures are in bijection with  .
.
For more information on 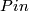 -manifolds, including a computation of the low-dimensional bordism groups
of
-manifolds, including a computation of the low-dimensional bordism groups
of 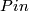 -manifolds, see [Kirby&Taylor1990].
-manifolds, see [Kirby&Taylor1990].
[edit] 2 Examples
In dimension  , all orientable surfaces admit a
, all orientable surfaces admit a  -structure, and hence both
-structure, and hence both 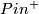 - and
- and 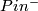 -structures. The nonorientable surface
-structures. The nonorientable surface 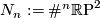 admits a
admits a 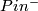 -structure, but admits a
-structure, but admits a 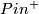 -structure if and only if
-structure if and only if  is even.
is even.
For 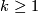 :
:
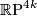 admits two
admits two 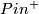 -structures and no
-structures and no 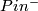 -structure.
-structure.
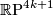 admits no
admits no 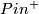 -structure and no
-structure and no 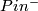 -structure.
-structure.
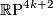 admits no
admits no 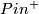 -structure and two
-structure and two 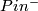 -structures.
-structures.
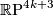 admits two
admits two 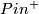 -structures and two
-structures and two 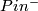 -structures.
-structures.
[edit] 3 References
- [Kirby&Taylor1990] R. C. Kirby and L. R. Taylor,
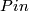 structures on low-dimensional manifolds, Geometry of low-dimensional manifolds, 2 (Durham, 1989), Cambridge Univ. Press (1990), 177–242. MR1171915 (94b:57031) Zbl 0754.57020
structures on low-dimensional manifolds, Geometry of low-dimensional manifolds, 2 (Durham, 1989), Cambridge Univ. Press (1990), 177–242. MR1171915 (94b:57031) Zbl 0754.57020