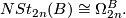Parametric connected sum
(→Connected sum) |
|||
| Line 2: | Line 2: | ||
== Introduction == | == Introduction == | ||
<wikitex>; | <wikitex>; | ||
| − | Parametric connected sum is an operation on compact connected n-manifolds $M$ and $N$ equipped with codimension 0-embeddings $\phi: T \to M$ and $\psi : T \to N$ of a compact connected manifold $T$. It generalises the usual connected sum operation | + | Parametric connected sum is an operation on compact connected n-manifolds $M$ and $N$ equipped with codimension 0-embeddings $\phi: T \to M$ and $\psi : T \to N$ of a compact connected manifold $T$. It generalises the usual [[Connected sum|connected sum]] operation |
| − | + | which is the special case when $T = D^n$ is the $n$-disc. | |
| − | + | The parametric connected sum operation is more complicated than the usual connected | |
| − | + | sum operation since the isotopy classes of the embeddings of $T$ into $M$ may be significantly more complicated than the isotopy classes of embeddings of n-discs need for connected sum: these last are determined by (local) orientations. | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
</wikitex> | </wikitex> | ||
| Line 62: | Line 48: | ||
<!-- --> | <!-- --> | ||
[[Category:Theory]] | [[Category:Theory]] | ||
| + | [[Category:Definitions]] | ||
Revision as of 18:06, 19 February 2013
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
Parametric connected sum is an operation on compact connected n-manifolds  and
and  equipped with codimension 0-embeddings
equipped with codimension 0-embeddings 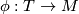 and
and 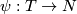 of a compact connected manifold
of a compact connected manifold  . It generalises the usual connected sum operation
which is the special case when
. It generalises the usual connected sum operation
which is the special case when 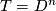 is the
is the  -disc.
The parametric connected sum operation is more complicated than the usual connected
sum operation since the isotopy classes of the embeddings of
-disc.
The parametric connected sum operation is more complicated than the usual connected
sum operation since the isotopy classes of the embeddings of  into
into  may be significantly more complicated than the isotopy classes of embeddings of n-discs need for connected sum: these last are determined by (local) orientations.
may be significantly more complicated than the isotopy classes of embeddings of n-discs need for connected sum: these last are determined by (local) orientations.
2 Connected sum along k-spheres
We say above that to define connected sum for connected k-manifolds  and
and  it is sufficient to equip them with an isotopy class of embeddings of the k-disc. Moreover, the disjoint union
it is sufficient to equip them with an isotopy class of embeddings of the k-disc. Moreover, the disjoint union  is the unique thickening of
is the unique thickening of 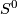 . This motivates the following
. This motivates the following
Defintion 2.1.
A manifold with an 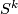 -thickening, an
-thickening, an 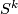 -thickened manifold for short, is a pair
-thickened manifold for short, is a pair 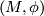 where
where  is a compact connected manifold and
is a compact connected manifold and 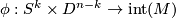 is an embedding.
is an embedding.
Defintion 2.2.
Let  and
and  by
by 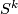 -thickened manifolds. Define
-thickened manifolds. Define
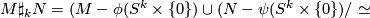
where 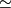 is defined via the embeddings
is defined via the embeddings  and
and 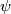 .
.
It is clear that we have the following
Observation 2.3.
The diffeomorphism type of 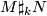 depends only upon the the isotopy classes of the embeddings
depends only upon the the isotopy classes of the embeddings  and
and 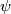 (which of course includes the diffeomorphism types of
(which of course includes the diffeomorphism types of  and
and  ).
).
2.1 Applications
The operation of 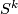 -connected sum was used in [Ajala1984] and [Ajala1987] to describe the set of smooth structures on the product of spheres
-connected sum was used in [Ajala1984] and [Ajala1987] to describe the set of smooth structures on the product of spheres 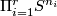 . This construction also appears in [Sako1981]. The analogue of such a construction for embeddings is used in [Skopenkov2006] to define, for
. This construction also appears in [Sako1981]. The analogue of such a construction for embeddings is used in [Skopenkov2006] to define, for 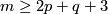 , a group stucture on the set
, a group stucture on the set 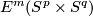 of (smooth or PL) isotopy classes of embeddings of
of (smooth or PL) isotopy classes of embeddings of 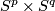 into
into  . In [Skopenkov2007] and [Skopenkov2010] the
. In [Skopenkov2007] and [Skopenkov2010] the 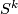 -connected sum of embeddings was used to estimate the set of embeddings.
-connected sum of embeddings was used to estimate the set of embeddings.
3 Parametric connected sum along thickenings
Let  be a stable fibred vector bundle. A foundational theorem of modified surgery is
be a stable fibred vector bundle. A foundational theorem of modified surgery is
In particular,  has the structure of an abelian group. The question of whether there is a geometric definition of this group structure is taken up in [Kreck1985, Chapter 2, pp 26-7] where it is shown how to use parametric connected sum along thickenings to define an addition of stable diffeomorphism classes of closed 2n-B-manifolds.
has the structure of an abelian group. The question of whether there is a geometric definition of this group structure is taken up in [Kreck1985, Chapter 2, pp 26-7] where it is shown how to use parametric connected sum along thickenings to define an addition of stable diffeomorphism classes of closed 2n-B-manifolds.
4 References
- [Ajala1984] S. O. Ajala, Differentiable structures on products of spheres, Houston J. Math. 10 (1984), no.1, 1–14. MR736571 (85c:57032) Zbl 0547.57026
- [Ajala1987] S. O. Ajala, Differentiable structures on a generalized product of spheres, Internat. J. Math. Math. Sci. 10 (1987), no.2, 217–226. MR886378 (88j:57028) Zbl 0627.57022
- [Kreck1985] M. Kreck, An extension of the results of Browder, Novikov and Wall about surgery on compact manifolds, preprint Mainz (1985).
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Sako1981] Y. Sako, Connected sum along the cycle operation of
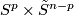 on
on  -manifolds, Proc. Japan Acad. Ser. A Math. Sci. 57 (1981), no.10, 499–502. MR640259 (83a:57043) Zbl 0505.57010
-manifolds, Proc. Japan Acad. Ser. A Math. Sci. 57 (1981), no.10, 499–502. MR640259 (83a:57043) Zbl 0505.57010
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2007] A. Skopenkov, A new invariant and parametric connected sum of embeddings, Fund. Math. 197 (2007), 253–269. arXiv:math/0509621. MR2365891 (2008k:57044) Zbl 1145.57019
- [Skopenkov2010] A. Skopenkov, Embeddings of k-connected n-manifolds into
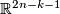 , Proc. AMS, 138 (2010) 3377--3389. Available at the arXiv:0812.0263.
, Proc. AMS, 138 (2010) 3377--3389. Available at the arXiv:0812.0263.