Hyperbolic Surfaces
m |
|||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{{Stub}} | {{Stub}} | ||
== Introduction == | == Introduction == | ||
| Line 51: | Line 39: | ||
... | ... | ||
</wikitex> | </wikitex> | ||
| − | <!-- | + | <!-- |
| − | + | ||
| − | + | ||
== Footnotes == | == Footnotes == | ||
<references/> --> | <references/> --> | ||
Latest revision as of 11:38, 13 June 2013
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
[edit] 1 Introduction
...
[edit] 2 Construction and examples
<a href="/File:Polygon_construction.png" class="internal" title="Enlarge"> <img src="/skins/common/images/magnify-clip.png" width="15" height="11" alt="" /> </a>
Any hyperbolic metric on a closed, orientable surface 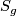 of genus
of genus 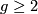 is obtained by the following
construction: choose a geodesic
is obtained by the following
construction: choose a geodesic 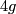 -gon in the hyperbolic plane
-gon in the hyperbolic plane 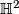 with area
with area 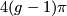 . (This implies that the sum of interior angles is
. (This implies that the sum of interior angles is 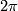 .) Then choose orientation-preserving isometries
.) Then choose orientation-preserving isometries 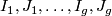 which realise the gluing pattern of
which realise the gluing pattern of 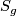 : for
: for 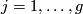 we require that
we require that 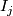 maps
maps 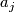 to
to 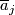 ,
, 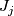 maps
maps 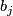 to
to 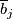 . Let
. Let 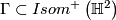 be the subgroup generated by
be the subgroup generated by 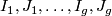 . Then
. Then 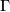 is a discrete subgroup of
is a discrete subgroup of 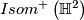 and
and 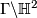 is a hyperbolic surface diffeomorphic to
is a hyperbolic surface diffeomorphic to 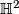 .
.
The moduli space of hyperbolic metrics on the closed, orientable surface 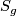 is
is 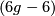 -dimensional.
-dimensional.
[edit] 3 Invariants
...
[edit] 4 Classification/Characterization
...
[edit] 5 Further discussion
...