Talk:Poincaré duality II (Ex)
From Manifold Atlas
- Note: There is a sign error in this solution to be fixed!! Give
 the triangulation with
the triangulation with  -simplices
-simplices 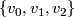 and
and  -simplices
-simplices ![\{[v_0,v_1], [v_1,v_2] , [v_2,v_0] \}](/images/math/b/8/9/b897f9ffebac9681294856527e8e56b7.png) . Let us choose the fundamental class
. Let us choose the fundamental class
![\displaystyle [S^1]=[v_0,v_1]\cup[v_1,v_2]\cup[v_2,v_0].](/images/math/8/b/e/8be16640591c92786323478fcaa75b5e.png)
The diagonal approximation  maps
maps ![[v_0,v_1]](/images/math/b/e/f/befc6b28c9768abda15aaf69d4a9891f.png) to
to
![\displaystyle \begin{aligned} && \sum_{p+q=1} {_p[v_0,v_1]\otimes [v_0,v_1]_q} \\&=& {_0[v_0,v_1]}\otimes [v_0,v_1]_1 + {_1[v_0,v_1]}\otimes [v_0,v_1]_0 \\&=& \{v_0\}\otimes [v_0,v_1] + [v_0,v_1]\otimes\{v_1\}.\end{aligned}](/images/math/6/8/7/687dca7cb07db58794cb5b952eef7984.png)
![\tau([S^1])](/images/math/0/d/8/0d88d6b0fef050415a6a49fed4e32a8f.png) is
is
![\displaystyle \{v_0\}\otimes [v_0,v_1] + [v_0,v_1]\otimes\{v_1\}+ \{v_1\}\otimes [v_1,v_2] + [v_1,v_2]\otimes\{v_2\} + \{v_2\}\otimes [v_2,v_0] + [v_2,v_0]\otimes\{v_0\}.](/images/math/7/2/7/72798d50329b17e88361a9823666b30b.png)
Capping with the fundamental class sends 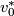 to
to
![\displaystyle \begin{aligned} && E(v_0^*\otimes\tau([S^1])) \\ &=& E(v_0^*\otimes ([v_2,v_0]\otimes\{v_0\}+ \mathrm{other}\;\mathrm{terms})) \\ &=& v_0^*(v_0)\otimes[v_2,v_0]\\ &=& [v_2,v_0]. \end{aligned}](/images/math/b/2/a/b2a9ab5aad4493a747f49000998165df.png)
Similarly, ![-\cap[S^1]](/images/math/8/f/8/8f8d98cbc57596c2795b7a5031858a2d.png) sends
sends
![\displaystyle \begin{aligned} v_1^* &\mapsto& [v_0,v_1], \\ v_2^* &\mapsto& [v_1,v_2], \\ [v_0,v_1]^* &\mapsto& v_0, \\ [v_1,v_2]^* &\mapsto& v_1, \\ [v_2,v_0]^* &\mapsto& v_2. \end{aligned}](/images/math/a/c/4/ac44303df7f47a1c7c73745c4744414a.png)
Thus we obtain the chain equivalence
![\displaystyle \xymatrix{ <v_0^*,v_1^*,v_2^*> = C^0(S^1)\ar[rr]^-{(-\cap[S^1])_1} \ar[d]_{\delta} && C_1(S^1)=<[v_0,v_1],[v_1,v_2],[v_2,v_0]> \ar[d]^{d} \\ <[v_0,v_1]^*,[v_1,v_2]^*,[v_2,v_0]^*>=C^1(S^1) \ar[rr]^-{(-\cap[S^1])_0} && C_0(S^1)=<v_0,v_1,v_2>}](/images/math/9/c/f/9cf2d64f51e5d44959662313d2f295f9.png)
Where
![\displaystyle (-\cap[S^1])_1 = \left( \begin{array}{ccc}0&1&0\\0&0&1\\1&0&0 \end{array}\right),\quad(-\cap[S^1])_0 = \left( \begin{array}{ccc}1&0&0\\0&1&0\\0&0&1 \end{array}\right),\quad d= \left( \begin{array}{ccc}-1&0&1\\1&-1&0\\0&1&-1 \end{array}\right),\quad \delta = \left( \begin{array}{ccc}-1&1&0\\0&-1&1\\1&0&-1 \end{array}\right).](/images/math/0/1/a/01abfe7816103fb5e9ceeb631efdd058.png)
Note: this doesn't quite work - this is not a chain map, composing one way gives minus the composition the other way. Fixing the sign error we can take the inverse permutation matrices as the chain inverse.