Talk:Microbundles (Ex)
First, You should get familiar with the definition of microbundle.
Exercise 0.1 [Milnor1964, Lemma 2.1].
Let  be a topological manifold. Show that
be a topological manifold. Show that 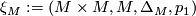 is a microbundle.
is a microbundle.
Proof.
Let  be a topological manifold. Then the composition
be a topological manifold. Then the composition 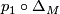 sends
sends 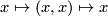 , so the first condition in the definition is satisfied.
, so the first condition in the definition is satisfied.
To prove that the second condition is satisfied we need to use local chart around  .
Choose
.
Choose  to be one of the open sets coming from atlas of
to be one of the open sets coming from atlas of  and let
and let 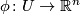 be associated chart. The obvious choice for neighbourhood
be associated chart. The obvious choice for neighbourhood  is to take
is to take  . The first naive candidate for
. The first naive candidate for 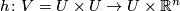 would be map
would be map 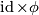 . However such
. However such  fails to make the following diagram commute
fails to make the following diagram commute
![\displaystyle \xymatrix{ &U\times U\ar[rd]^{p_1}\ar[dd]^h&\\ U\ar[ru]^{\Delta_M}\ar[rd]_{\id\times\{0\}} & & U\\ &U\times \Rr^n\ar[ru]_{p_1}&}](/images/math/4/c/d/4cd938113e2094115097195f965b3b01.png)
since 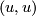 is mapped to
is mapped to 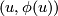 and
and  doesn't necessarily be
doesn't necessarily be  (well, it depends on our definition of an atlas of a manifold?). We need just a small adjustment:
(well, it depends on our definition of an atlas of a manifold?). We need just a small adjustment: 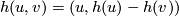 .
.

Exercise 0.2 [Milnor1964, Theorem 2.2].
Let  be a (paracompact!) smooth manifold. Show that
be a (paracompact!) smooth manifold. Show that  and
and 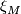 are isomorphic microbundles.
are isomorphic microbundles.
Proof.
We have two concurring definitions of micro-tangent bundle. The first is given by the exercise above, the second by forgeting about the vector bundle structure on  and treating it just as a microbundle
and treating it just as a microbundle 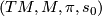 where
where 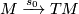 is the zero section.
is the zero section.
To fix the notation please consult the definition of microbundle isomorphism on page on microbundles .
In our case we have
![\displaystyle \xymatrix{ & V\ar[dd]^H \ar[rd]^{\pi}&\\ M\ar[dr]_{\Delta_M}\ar[ur]^{s_0} & & M\\ & M\times M \ar[ru]_{p_1}&}](/images/math/b/6/3/b630c92c98dd6c12008a067c2eee3046.png)
where 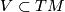 is an open neighbourhood of the zero section.
is an open neighbourhood of the zero section.
We need to find a neighbourhood  and a map
and a map 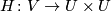 such that points in the zero section (
such that points in the zero section (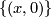 in local coordinates) are mapped to the diagonal
in local coordinates) are mapped to the diagonal 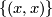 .
.
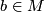 and let
and let 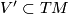 be a neighbourhood of
be a neighbourhood of  coming from the vector bundle structure. Choose a trivialization
coming from the vector bundle structure. Choose a trivialization 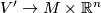 and then set
and then set 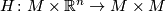 ,
, 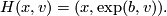
 we have
we have 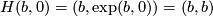 .
However, we may now let
.
However, we may now let  vary as
vary as  does and define
does and define 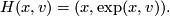
 is non-vanishing along the zero section, so by the inverse function theorem there exist a neighbourhood
is non-vanishing along the zero section, so by the inverse function theorem there exist a neighbourhood 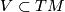 of
of  on which
on which  is a diffeomorphism.
is a diffeomorphism.
