Novikov Conjecture
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Introduction
Let  be a closed oriented
be a closed oriented  -dimensional smooth manifold with a map
-dimensional smooth manifold with a map 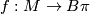 for some discrete group
for some discrete group  and let
and let  be a rational cohomology class. The higher signature of
be a rational cohomology class. The higher signature of  defined by
defined by 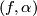 is the rational number
is the rational number
![\displaystyle \sigma_\alpha(M, f) = \langle L_M \cup f^*\alpha, [M] \rangle \in \Qq](/images/math/9/1/4/9143205dcee6fec1293e14ba2f0abe7f.png)
where  is the Hirzebruch L-class of
is the Hirzebruch L-class of  . Let
. Let 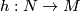 be a homotopy equivalence of closed oriented smooth manifolds. The Novikov conjecture states
be a homotopy equivalence of closed oriented smooth manifolds. The Novikov conjecture states
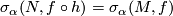
for all  ,
,  ,
,  and for all homotopy equivalences
and for all homotopy equivalences 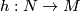 . For the trivial group
. For the trivial group  the conjecture is true by Hirzebruch's signature theorem.
the conjecture is true by Hirzebruch's signature theorem.
The original 1969 statement of the Novikov conjecture may be found in [Novikov1970] and [Novikov1970a]: a history and survey including the original statement in Russian with a translation into English may be found in [Ferry&Ranicki&Rosenberg1995b]. In the last 40 years the Novikov conjecture and the related conjectures of Borel and Farrell-Jones have been the subject of a great deal of research. In [Novikov2010] Novikov described how he came to formulate the conjecture.
More information may be found in [Ferry&Ranicki&Rosenberg1995], [Ferry&Ranicki&Rosenberg1995a], [Ranicki1995] and [Davis2000].
[edit] 2 References
- [Davis2000] J. F. Davis, Manifold aspects of the Novikov conjecture, Surveys on surgery theory, Vol. 1, Princeton Univ. Press (2000), 195–224. MR1747536 (2002a:57037) Zbl 0948.57001
- [Ferry&Ranicki&Rosenberg1995] S. C. Ferry, A. A. Ranicki and J. Rosenberg, Novikov conjectures, index theorems and rigidity. Vol. 1. London Math. Soc. Lecture Note Ser., 226, Cambridge Univ. Press, Cambridge, 1995. MR1388294 (96m:57002) Zbl 0829.00027
- [Ferry&Ranicki&Rosenberg1995a] S. C. Ferry, A. A. Ranicki and J. Rosenberg, Novikov conjectures, index theorems and rigidity. Vol. 2, London Math. Soc. Lecture Note Ser., 227, Cambridge Univ. Press, Cambridge, 1995. MR1388306 (96m:57003) Zbl 0829.00028
- [Ferry&Ranicki&Rosenberg1995b] S. C. Ferry, A. A. Ranicki and J. Rosenburg, A history and survey of the Novikov conjecture in Ferry&Ranicki&Rosenberg1995 7–66, London Math. Soc. Lecture Note Ser., 226, Cambridge Univ. Press, Cambridge, 1995. MR1388295 (97f:57036) Zbl 0954.57018
- [Novikov1970] S. P. Novikov, Algebraic construction and properties of Hermitian analogs of
 -theory over rings with involution from the viewpoint of Hamiltonian formalism. Applications to differential topology and the theory of characteristic classes. I. II, Math. USSR-Izv. 4 (1970), 257–292; ibid. 4 (1970), 479–505; translated from Izv. Akad. Nauk SSSR Ser. Mat. 34 (1970), 253–288; ibid. 34 (1970), 475. MR0292913 (45 #1994) Zbl 0216.45003 Zbl 0233.57009
-theory over rings with involution from the viewpoint of Hamiltonian formalism. Applications to differential topology and the theory of characteristic classes. I. II, Math. USSR-Izv. 4 (1970), 257–292; ibid. 4 (1970), 479–505; translated from Izv. Akad. Nauk SSSR Ser. Mat. 34 (1970), 253–288; ibid. 34 (1970), 475. MR0292913 (45 #1994) Zbl 0216.45003 Zbl 0233.57009
- [Novikov1970a] S. P. Novikov, Pontrjagin classes, the fundamental group and some problems of stable algebra 1970 Essays on Topology and Related Topics (Mémoires dédiés à Georges de Rham) pp. 147–155 Springer, New York. MR0268907 (42 #3804)
- [Novikov2010] S. P. Novikov, Scholarpedia article on the Novikov Conjecture (2010)
- [Ranicki1995] A. A. Ranicki On the Novikov conjecture in [Ferry&Ranicki&Rosenburg1995], 272–337, London Math. Soc. Lecture Note Ser., 226, Cambridge Univ. Press, Cambridge, 1995. MR1388304 (97d:57045) Zbl 0954.57017
[edit] 3 External links
- The Wikipedia page about the Novikov conjecture.