Kappa classes (Ex)

Let $i \colon SG \to G/TOP$$\newcommand{\Z}{\mathbb{Z}} \newcommand{\R}{\mathbb{R}} \newcommand{\C}{\mathbb{C}} \newcommand{\N}{\mathbb{N}} \newcommand{\Q}{\mathbb{Q}} \newcommand{\F}{\mathbb{F}} \newcommand{\bZ}{\mathbb{Z}} \newcommand{\bR}{\mathbb{R}} \newcommand{\bC}{\mathbb{C}} \newcommand{\bH}{\mathbb{H}} \newcommand{\bQ}{\mathbb{Q}} \newcommand{\bF}{\mathbb{F}} \newcommand{\bN}{\mathbb{N}} \DeclareMathOperator\id{id} % identity map \DeclareMathOperator\Sq{Sq} % Steenrod squares \DeclareMathOperator\Homeo{Homeo} % group of homeomorphisms of a topoloical space \DeclareMathOperator\Diff{Diff} % group of diffeomorphisms of a smooth manifold \DeclareMathOperator\SDiff{SDiff} % diffeomorphism under some constraint \DeclareMathOperator\Hom{Hom} % homomrphism group \DeclareMathOperator\End{End} % endomorphism group \DeclareMathOperator\Aut{Aut} % automorphism group \DeclareMathOperator\Inn{Inn} % inner automorphisms \DeclareMathOperator\Out{Out} % outer automorphism group \DeclareMathOperator\vol{vol} % volume \newcommand{\GL}{\text{GL}} % general linear group \newcommand{\SL}{\text{SL}} % special linear group \newcommand{\SO}{\text{SO}} % special orthogonal group \newcommand{\O}{\text{O}} % orthogonal group \newcommand{\SU}{\text{SU}} % special unitary group \newcommand{\Spin}{\text{Spin}} % Spin group \newcommand{\RP}{\Rr\mathrm P} % real projective space \newcommand{\CP}{\Cc\mathrm P} % complex projective space \newcommand{\HP}{\Hh\mathrm P} % quaternionic projective space \newcommand{\Top}{\mathrm{Top}} % topological category \newcommand{\PL}{\mathrm{PL}} % piecewise linear category \newcommand{\Cat}{\mathrm{Cat}} % any category \newcommand{\KS}{\text{KS}} % Kirby-Siebenmann class \newcommand{\Hud}{\text{Hud}} % Hudson torus \newcommand{\Ker}{\text{Ker}} % Kernel \newcommand{\underbar}{\underline} %Classifying Spaces for Families of Subgroups \newcommand{\textup}{\text} \newcommand{\sp}{^}i \colon SG \to G/TOP$ 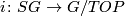be the canonical map and let 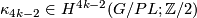$\kappa_{4k-2} \in H^{4k-2}(G/PL; \Zz/2)$$\kappa_{4k-2} \in H^{4k-2}(G/PL; \Zz/2)$ be defined as in [Madsen&Milgram1979, Theorem 4.9]. 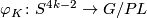In addition let $\varphi_K \colon S^{4k-2} \to G/PL$$\varphi_K \colon S^{4k-2} \to G/PL$ be the normal invariant of a degree one normal map 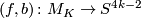$(f, b) \colon M_K \to S^{4k-2}$$(f, b) \colon M_K \to S^{4k-2}$ from the Kervaire manifold to the 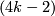$(4k-2)$$(4k-2)$-sphere.

Exercise 0.1.

1. Show that 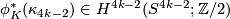$\phi_K^*(\kappa_{4k-2}) \in H^{4k-2}(S^{4k-2}; \Zz/2)$$\phi_K^*(\kappa_{4k-2}) \in H^{4k-2}(S^{4k-2}; \Zz/2)$ is the generator.
2. Show that there is a framed manifold of Kervaire invariant one in dimension 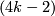$(4k-2)$$(4k-2)$ if and only if there is a map 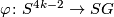$\varphi \colon S^{4k-2} \to SG$$\varphi \colon S^{4k-2} \to SG$ such that 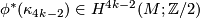$\phi^*(\kappa_{4k-2}) \in H^{4k-2}(M; \Zz/2)$$\phi^*(\kappa_{4k-2}) \in H^{4k-2}(M; \Zz/2)$ is a generator.
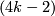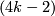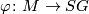3. Show that there is a framed manifold of Kervaire invariant one in dimension $(4k-2)$$(4k-2)$ if and only if there is a closed framed $(4k-2)$$(4k-2)$-manifold $M$$M$ and a map $\varphi \colon M \to SG$$\varphi \colon M \to SG$ such that 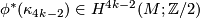$\phi^*(\kappa_{4k-2}) \in H^{4k-2}(M; \Zz/2)$$\phi^*(\kappa_{4k-2}) \in H^{4k-2}(M; \Zz/2)$ is a generator.

References

• [Madsen&Milgram1979] I. Madsen and R. J. Milgram, The classifying spaces for surgery and cobordism of manifolds, Princeton University Press, Princeton, N.J., 1979. MR548575 (81b:57014) Zbl 0446.57002